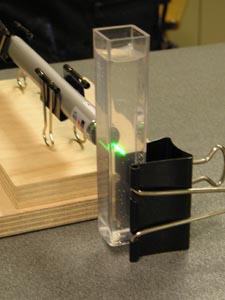
A laser shines through a dilute milk solution.
Introduction
Shine a laser through a dilute milk solution and observe the changing pattern of dots in the scattered light.

A laser shines through a dilute milk solution.
Material
Assembly
Fill a container with water
Add a drop of milk or a pinch of dry milk to the water.
The container should be clear enough after the milk is added to allow a newspaper to be read through the container.
To Do and Notice
Shine the laser through the container of milky water onto a white screen.
The laser should be mounted so it does not move.
The screen should be moved away until the pattern of scattered light is large but still visible.
Notice the pattern of light and dark on the screen surrounding the laser dot.
Notice how the pattern changes with time. The pattern changes slowly near the laser dot, the pattern changes more rapidly farther from the dot.
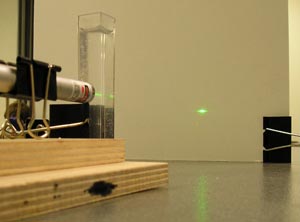
The diffraction pattern from laser light shone through a dilute milk
solution makes a twinkling pattern on a screen.
You can also see the twinkling by looking at the laser light scattered in the forward direction by the milk solution.
Another way to show the twinkling light to an audience is to project it onto a translucent screen such as one made from wax paper.

The diffraction pattern from a milk solution viewed in a wax
paper screen.
What's Going On?
The pattern of scattered light changes because the tiny balls of fat in the milk are in motion.
The balls move in Brownian Motion, a random walk driven by collisions with water molecules.
The light scattered by the small balls of milk fat is a diffraction pattern.
Diffraction at large angles is produced by small
spacings between scatterers,
diffraction through small angles is created by large spacings between
scatterers.
See the activity Laser
Diffraction.
It takes a long time for milk balls to move through large distances to change the small angle scattering, it takes short times for them to move through small distances to change the pattern at larger angles.
So the pattern changes slowly near the laser dot and changes more rapidly farther away.
Math Root
In 1905, Albert Einstein was the first person to provide a mathematical analysis of Brownian motion.
He showed that particles undergoing Brownian motion followed a random walk and that the distance, x, that a particle moved from an initial position was proportional to the square root of the time, with a constant of proportionality known as the diffusion coefficient, D.
x = (Dt)^0.5
or x^2 = Dt
where D has units of m^2/s.
The pattern of twinkling in the diffraction pattern of laser light through milk follows Einstein's prediction.
Einstein also showed the relationship between the smaller atoms and molecules that were constantly colliding with the fat blobs in the milk causing them to undergo a random walk.
When the Brownian motion of small particles was analyzed by Perrin he was able to calculate the sizes of atoms.
Perrin's work based on Einstein's mathematical analysis convinced many scientists of the reality of atoms.
Etc.
Water molecules collide with the milk fat balls 10^14 times per second.
|
Scientific Explorations with Paul Doherty |
|
18 August 2005 |