Interference colors in a soap film
Introduction
Here is a model of wave addition which will guide you in exploring the colors of light reflected by soap bubbles.
Material
Assembly
Use a blue pen to draw a single sinewave on twenty-four 3x5 cards. The wave should start at the left center of the card and go up to a maximum.
Flip the cards over top-to-bottom and draw a wave on the back which starts down from the center of the left edge. (If you hold the card up to a light, the wave on the front and the wave on the back will coincide.)
Use a red pen to draw similar waves on the larger
cards.
Tape the 3x5 cards together in rows of 12 cards.
Tape the 5 x 7 cards together in rows of 8 cards.
Teacher suggestion: To draw more accurate sine waves draw a line down the center of each card, mark the mid point. also mark a point along the top of the card 1/4 way from the left edge and a point on the bottom a quarter of the way from the right edge. Draw the sinwave starting on the center line at the left through the top, center and bottom points ending on the right center.
To Do and Notice
The colors of soap films.
To see the colors of soap films do the activity Soap Film in a Can.
Start using the smaller, blue cards.
Make two parallel lines with masking tape on the floor.
Make the lines one blue wavelength apart (For index cards 5 inches or
12.5 cm, for acetate strips 2.75 inches or 6.5 cm)
Start two waves,with at least two maxima to the
left of the soap film.
Adjust their position so that they strike the soap film surfaces at
the maximum of each wave.
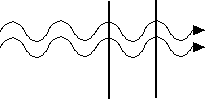
Light waves from the left hit the two sides of a soap film
The reflection from the back of the soap film can be found by simply folding the wave back on itself along the line representing the back of the bubble. The drawing on the back of the index cards then accurately shows the reflected wave. When you do this the outgoing and incoming wave lay exactly on top of each other. (The exact alignment of incoming and outgoing waves is only true when you position the maximum or a minimum at the reflecting surface.)
When a light wave reflects as it goes from a high speed of light material (air) to a lower speed of light material (soap) the wave flips over. To make the beam of light reflected by the first surface flip over, flip the wave about a horizontal axis (top-to-bottom) so that the maximum becomes a minimum, then fold the wave back on itself along the line representing the front surface of the soap film.

The reflected waves from the two surfaces are out
of phase when they combine and so cancel.
What’s Going On?
There are two contributions to the phase of the reflected waves from the two surfaces of soap films: the wave flips over as it is reflected off the front surface, that is, as light goes from air to soap, and the extra path length traveled by the wave which goes into the soap then reflects off the back surface and returns to the front. When the soap film is thin, there is little contribution from the extra path length and so the waves add out-of-phase and cancel. There is no reflection from such a thin bubble. Since the colors of bubbles are usually viewed against a black background, this thin bubble with no reflection is called “black” even though it is actually transparent.
Aside
The up and down of the wave represents the electric forces of the light wave on a test charge. When a wave bounces off the back of the film the part that just hit the film is nearest to the film, while the part that hit a while ago is further away. Folding the wave back on itself creates exactly the pattern needed to show the forces on a charge from the light reflected from the back of the film without inversion. Flipping the wave over top-to-bottom and then folding it back on itself gives the forces after inversion. The test charge feels the sum of the two forces one due to the reflection off the front surface, one due to reflection off the back.
It’s more complicated than that.
The wavelength of the light actually changes when it enters the soap film. In our model we have not added this additional complication. Think of the thickness of the soap film in terms of the number of wavelengths measured in the soap film. Since the index of refraction of soap is a little higher than that of water, for soap use n = 1.4, then the wavelength of light in soap is the wavelength in air divided by 1.4.
To Do and Notice
Explore what happens when the soap films are very close together. One of the waves flips over, and there is no additional phase shift because there is no extra path length. the waves cancel.
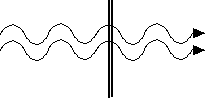
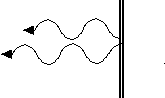
In a thin film, the reflections off the front and the back cancel.
To Do and Notice
Explore what happens when the soap films are 1/2 wavelength apart so that the front surface is at a wave maximum while the back is at a minimum. The extra pathlength is one full wave, so the waves cancel.
So far, it looks like light is never reflected
from soap films. However, try a soap film thickness of 1/4
wavelength. Align the front of the soap film with the maximum of the
waves the the back will then be at a zero crossing.
Flip over the wave reflected from the front before you fold it back
on itself.
Just fold back on itself the wave that hits the back.
The reflected wave from the back will add with the reflection from
the front surface in-phase and make a stronger reflection.
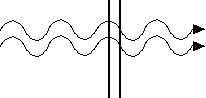
What’s Going On?
The reflection from the front surface is flipped over, the reflection from the back travels a half wavelength extra so it arrives at the front surface with the same phase and so adds to the front surface reflection.
To Do and Notice
Explore soap films which are 1/4 and 1/2 of a blue wavelength in thickness a second time using red light. Notice that where the soap film reflections cancel for blue light they add for red light and vice versa.
Further Explorations
To Do and Notice
To explore any thickness of soap film use the
following recipe.
Always arrange the incoming light so that a maximum of each wave is
at the front surface of the soap film.
Flip over top to bottom the wave that hits the front surface.
Fold the wave that hits the back surface back on itself.
Add the two waves together as they exit the film.
What's Going On?
When the wave reflects off the back surface without inversion what reflects off is what has just hit the surface. If the part of the sinewave which crosses zero in a downward direction has hit the back surface a short while ago and passed on through the film, then this same downward zero crossing will have reflected off the film and propagated toward the front of the film. This is simply accomplished by folding the wave back on itself.
Additional material
Assembly
Cut the acetate sheets into 4 long strips (11
inches long and 2.1 inches wide)
Draw two red sinewaves along the 11 inch length of 8 acetate
strips.
Draw 4 blue sinewaves along the 11 inch length of 8 acetate
strips.
Use the transparent tape to tape the strips together.
Tape 4 strips together so that their waves match up into one long
wave.
Continue with the exploration in the same way as with the 3x5 cards. You can now use an overhead projector.
Etc
Soap bubbles have two different stable thicknesses which look black. The thicker of these is called the common black film, it is 30 nm thick &emdash; about 300 atoms thick or 10 soap molecules or 1/20 the wavelength of light. The thinner film is called the Newton black film, it is about 6 nm thick,1/100 the wavelength of light, or two soap molecules, and is much more transparent, i.e. much “blacker.”
Math Root
One complete sine wave has a phase associated with
it of 2p
radians, (p
= pi).
The phase difference between two waves which are in-phase is 0,
2p, or
any even multiple of p
radians.
Waves which completely cancel have a phase difference of
p,
3p, or
any odd multiple of p
radians.
Waves with in-between phase differences add together with amplitudes
between those of completely in-phase and completely out-of-phase.
Reflections from the surfaces of bubbles which are thin compared to a
wavelength of light have a phase difference of
p from
the flipping over of the reflection from the front surface, there is
little phase difference from the extra path length.
Reflections from bubbles one half-wavelength thick have a total phase
difference of 3p;
one p
from the flipping over at the front surface, and two more from the
extra path length which is two half waves or one complete wave.
Reflections from bubbles which are 1/4 wavelength thick have a total
phase difference of 2p,
one p
from the flipping over at the front surface, the second from the
extra half wave the other reflection travels through the film and
back.
Math Root 2
The fraction of the energy of the light reflected
at the first surface is 4% of the incoming beam (when the light comes
into the soap film nearly perpendicular). We can measure this
reflection from single surface of soap, such as occur at the top of
vats of soap.
The energy reflected is the square of the amplitude of the light
wave. So if the light wave has an amplitude of 1.0 then the reflected
light has an amplitude of 0.2. The intensity of the reflected light
is the amplitude squared or 0.22 =
0.04.
To find the intensity of the reflected light we must add the
amplitudes of the waves we have been modeling and then square the
results. So when the light reflects in phase from two surfaces we add
the two amplitudes, 0.2 + 0.2 = 0.4 and then square to find that 0.16
or 16% of the incident light is reflected by a two surface soap film
which is 1/4 wavelength thick.
Many people expect that a two surface soap film will reflect twice as
much as a single soap film or 8%, when the reflected waves have a
constant phase difference, this expectation is incorrect.
First you add the reflected amplitudes and then you square the result to find the resulting intensity of the scattered light.
Going Further
You can see thin film interference colors in the exploration Permanent Oil Slick.
Scientific Explorations
with Paul Doherty 24 May 2000